One to one maths interventions built for KS4 success
Weekly online one to one GCSE maths revision lessons now available
In order to access this I need to be confident with:
Place value Arithmetic Laws of indices Negative numbersThis topic is relevant for:

Standard Form
Here we will learn about standard form including how to convert between ordinary numbers and standard form, and how to calculate with numbers in standard form.
There are also standard form worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.
What is standard form?
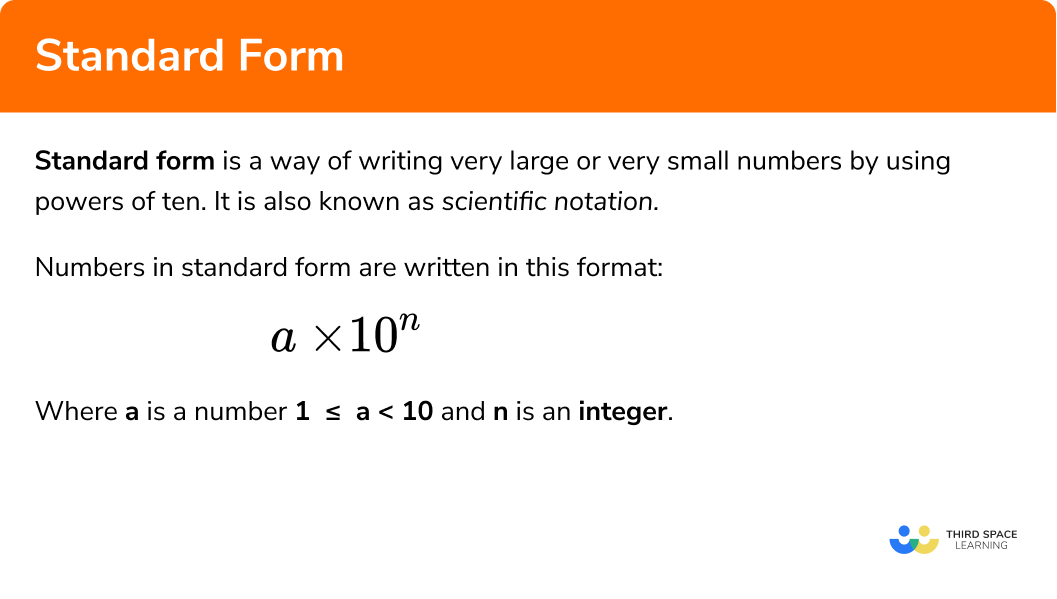
Standard form is a way of writing very large or very small numbers by comparing the powers of ten. It is also known as scientific notation.
Numbers in standard form are written in this format:
Where
To do this we need to understand the place value of a number
E.g.
Let’s look at the number
So
What is standard form?
Standard form notation
Standard form notation is a representation of place value which compliments the decimal number system, as shown in the table below.
| Words | Decimal form | Fraction form | Fraction form with powers of ten | Powers of ten | Standard form |
| Thousand | 1000 | 1000 | 10^3 | 10^3 | 1\times10^{3} |
| Hundred | 100 | 100 | 10^2 | 10^2 | 1\times10^{2} |
| Ten | 10 | 10 | 10^1 | 10^1 | 1\times10^{1} |
| Unit | 1 | 1 | 10^0 | 10^0 | 1\times10^{0} |
| Tenth | 0.1 | \frac{1}{10} | \frac{1}{10^1} | 10^{-1} | 1\times10^{-1} |
| Hundredth | 0.01 | \frac{1}{100} | \frac{1}{10^2} | 10^{-2} | 1\times10^{-2} |
| Thousand-th | 0.001 | \frac{1}{1000} | \frac{1}{10^3} | 10^{-3} | 1\times10^{-3} |
Any integer or terminating decimal can be written using the standard form notation a\times10^{n}. The table below shows how the value of a can remain the same while the power of ten ‘n’ changes the place value of those digits.
| Decimal form | Standard form |
| 4500 | 4.5\times10^{3} |
| 450 | 4.5\times10^{2} |
| 45 | 4.5\times10^{1} |
| 4.5 | 4.5\times10^{0} |
| 0.45 | 4.5\times10^{-1} |
| 0.045 | 4.5\times10^{-2} |
| 0.0045 | 4.5\times10^{-3} |
Using standard form notation enables us to write very large or very small numbers.
E.g.
67 500 000 000 000 000 000 000 = 6.75\times10^{22} 0.000 000 000 000 037 = 3.7\times10^{-14}Using standard form notation also enables us to compare the size of very large or very small numbers easily.
E.g.
Which is larger, 8560000000000 or 45320000000000?
At a glance it is difficult to tell which is larger, but written in standard form you can compare these numbers very quickly as shown.
Which is larger, 8.56\times10^{12} or 4.532\times10^{13}?
Instantly we can see that 4.532\times10^{13} is the larger number as it has the higher power of ten.
Standard form calculation
When numbers are written in standard form it can make some calculations neater and quicker to compute. This is particularly the case for calculations involving multiplication, division and powers. However, this is reliant on an understanding of the rules of indices.
Multiplication rule a^{b} \times a^{c} = a^{b+c}
Division rule a^{b} \div a^{c} = a^{b-c}
Zero power rule a^{0} = 1
Power of power rule (a^{b})^{c} = a^{b \times c}
To review this topic see step-by-step guide: Laws of indices
E.g.
340000 \times 0.002
In standard form this changes to (3.4 \times 10^{5}) \times (2 \times 10^{-3})
Brackets are often used to show each separate number in standard form but they are not actually necessary 3.4 \times 10^{5} \times 2 \times 10^{-3}
As multiplication is commutative (the order does not matter) we can reorder this calculation to 3.4 \times 2 \times 10^{5} \times 10^{-3}
3.4 \times 2 = 6.8
10^{5} \times 10^{-3} = 10^{5+-3} = 10^{2} (applying the multiplication rule of indices a^{b} \times a^{c} = a^{b+c})
(3.4 \times 10^{5}) \times (2 \times 10^{-3}) = 6.8 \times 10^{2}
340000 \times 0.002 = 680
Let’s look at how to calculate with standard form in more detail.
How to calculate with standard form
In order to write numbers in standard form
- Identify the non-zero digits and write these as a decimal number which is greater than or equal to
1 but less than10 - In order to maintain the place value of the number, this decimal number needs to be multiplied by a power of ten
- Write the power of ten as an exponent
- Write your number in standard form
E.g.
Convert
- Writing
4500 as a decimal between1 and10 is4.5 4.5 × 1000 103 = 1000 - So
4500 written in standard form is4.5 × 103
In order to convert from standard form to ordinary numbers
- Convert the power of ten to an ordinary number
- Multiply the decimal number by this power of ten
- Write your number as an ordinary number
E.g.
Convert
104 = 10000 7.1 × 10000 - So
7.1 × 104 as an ordinary number is71000
Step-by step guide: Converting to and from standard form
In order to multiply and divide in standard form
- Multiply or divide the non-zero numbers
- Multiply or divide the powers of ten by adding or subtracting the indices
- Write the solution in standard form, checking that the first part of the number is
1 ≤x <10
E.g.
Work out
4 × 2 = 8 105 × 108 = 1013 8 × 1013 is in standard form
E.g.
Work out
2 ÷ 4 = 0.5 103 ÷ 108 = 10-5 0.5 × 10-5 is not in standard form
To compensate, you need to divide the power of ten by
So the answer is
Step-by-step guide: Multiplying and dividing in standard form
In order to add and subtract numbers in standard form
- Convert the number with the lower power of ten so that both numbers have the same power of ten
- Add the non-zero integers
- Check your answer is in standard form
E.g.
6 × 104 = 0.6 × 105 3 + 0.6 = 3.6 3.6 × 105 is in standard form
E.g.
3 × 103 = 0.3 × 104 9 − 0.3 = 8.7 8.7 × 104 is in standard form
Step-by-step guide: Adding and subtracting in standard form
Explain how to calculate with standard form


Standard form worksheet

Get your free standard form worksheet of 20+ questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREE
Standard form worksheet

Get your free standard form worksheet of 20+ questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREEStandard form examples
Example 1: writing numbers in standard form
Write this number in standard form:
52000
- The non-zero digits need to be written as a decimal number
The number needs to lie between 1≤ x <10
So the number will begin as 5.2…
2You now need to maintain the value of the number by multiplying that decimal by a power of ten
3Write that power of ten as an exponent
4Write your number in standard form
Example 2: writing a number in standard form as an ordinary number
Write 9.4\times10^5 as an ordinary number.
Write the exponent as a power of ten
Multiply the decimal number by that power of ten
Write your answer as an ordinary number
Example 3: writing a small number in standard form
Write 0.0068 in standard form.
The non-zero digits need to be written as a decimal number. The number needs to lie between 1≤ x <10
So the number will begin as
Identify what power of ten the decimal needs to be multiplied by in order to preserve place value
Write that power of ten as an exponent
Write your number in standard form
Example 4: multiplying numbers in standard form
Calculate (5\times10^{4}) \times (7\times10^{8}) .
Write your answer in standard form.
Multiply the non-zero digits
Multiply the powers of ten by adding the powers
Put these two parts together
However, this number is not in standard form as 35 is not a decimal number.
To convert
To maintain the value of the number, you need to multiply the power of ten by 10, which adds one to the exponent.
Example 5: dividing numbers in standard form
Calculate (8\times10^{7})\div(2\times10^{2}) .
Write your answer in standard form.
Divide the non-zero digits
Divide the powers of ten by subtracting the powers
Put these two parts together
Check your number is in standard form
This is in standard form as 4 is greater than 1 but less than 10.
Example 6: adding numbers in standard form
Calculate (6\times10^{4})+(2\times10^{3}) .
Write your answer in standard form.
Convert the number with the smaller power so that both numbers have the same power
You need to increase the power, to do this multiply it by
You will now have two numbers with the same exponent
6\times10^4 and 0.2\times10^4
You now add the two non-zero numbers
The exponents are the same after what you did in step 1
So your answer in standard form is 6.2\times10^4 .
Example 7: subtracting numbers in standard form
Calculate (5\times10^{4})−(4\times10^{3}) .
Write your answer in standard form.
Convert the number with the smaller power so that both numbers have the same power
To do this identify the number with the lowest exponent.
You need to increase the power. To do this multiply it by 10 to add one to the power. But to maintain the value of the number you need to divide the non-zero number by 10.
You will now have two numbers with the same exponent
5\times10^{4} and 0.4\times10^{4}
You now add the two non-zero numbers
The exponents are the same after what you did in step 1
So your answer in standard form is 4.6\times10^4 .
Common misconceptions
- Writing a number with the incorrect power for a large or small number
This can happen by counting the zeros following the first non zero digit for large numbers or zeros after the decimal point for small numbers, then writing this as the power.
- Identifying incorrect place value with small numbers
For example, in a number such as
- Not converting solutions to standard form
After calculating with standard form, a common mistake is to not check that the first part of the number is
- Not giving the solution in the correct form
It is important to check what form the question asks for the solution in – ordinary number or standard form.
Practice standard form questions
1. Write 86,000 in standard form.




The number between 1 and 10 here is 8.6. Since 8 is in the ten thousands column,
\begin{aligned} 86000&=8.6 \times 10000\\\\ &= 8.6 \times 10^{4} \end{aligned}
2. Write 0.0097 in standard form.




The number between 1 and 10 here is 9.7. Since 9 is in the thousandths position,
\begin{aligned} 0.0097 &= 9.7 \times \frac{1}{1000}\\\\ &= 9.7 \times 10^{-3} \end {aligned}
3. Write 5.9 \times 10^{3} as an ordinary number.




Therefore
\begin{aligned} 5.9 \times 10^{3} &= 5.9 \times 1000\\\\ &=5900 \end{aligned}
4. Work out (6\times10^{8})\times(3\times10^{4}) . Write your answer in standard form.




Therefore
(6 \times 10^{8}) \times (3 \times 10^{4}) = 18 \times 10^{12}
However 18 \times 10^{12} is not in standard form since 18 is not between 1 and 10.
So we need to divide 18 by 10 and, to compensate, increase the power of ten by 1.
This gives us
1.8 \times 10^{13}
5. Work out (9\times10^{7}) \div (4\times10^{2}) . Write your answer in standard form.




Therefore
(9 \times 10^{7}) \div (4 \times 10^{2}) = 2.25 \times 10^{5}
This is already in standard form since
1 \leq 2.25 <10
6. Work out (7\times10^{5})+(2\times10^{4}) . Write your answer in standard form.




(increase the power of ten by 1 and divide the 2 by 10 to compensate)
(7.2 \times 10^{5}) + ( 0.2 \times 10^{5}) = 7.2 \times 10^{5}
Standard form GCSE questions
1. The distance from Earth to Mars is 2.88\times10^8km, to three significant figures. Write this number in standard form.
(1 Mark)
288, 000, 000 km
(1)
2. (a) The speed of light is 3\times10^8m/s , rounded to one significant figure. Write this as an ordinary number.
(b) The mass of Earth is 5.97\times10^{24}kg . Work out the mass of the Earth in grams. Write your answer in standard form.
(3 Marks)
(a)
300, 000, 000 m/s
(1)
(b)
Recognising 1000g in a kilogram or multiplying by 1\times10^3
(1)
5.97\times10^{27} g
(1)
3. Put these numbers in order. Start with the smallest number.
62\times10^{-2}, \quad \quad 0.0068, \quad \quad 6.9\times10^{-3}, \quad \quad 0.0607
(2 Marks)
Converting all of the numbers to the same form for comparison or orders 3 of the four numbers correctly.
(1)
0.0068, \quad \quad 6.9\times10^{-3}, \quad \quad 0.0607, \quad \quad 62\times10^{-2}
(1)
Learning checklist
You have now learned how to:
- Write numbers in standard form
- Calculate with numbers in standard form
The next lessons are
Still stuck?
Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.
Find out more about our GCSE maths tuition programme.